Developing Fine Motor Skills: Dodecahedron

This material is suitable for children of any school age.

These developments are suitable for those who teach or want to teach a visually impaired child mathematics.

Each person has a unique way of perceiving information. People perceive information through multiple channels simultaneously, each of which transmits diverse data.

For understanding and memorizing new information, it is necessary to activate all channels of perception. “A child is not a vase to be filled, but a fire to be lit” (François Rabelais).

The most important senses for perceiving the surrounding world in visually impaired children are touch and hearing.

One way to develop spatial representations is by modeling and creating visual aids.

Lesson purpose: To develop skills to tactilely explore objects with different surfaces, and to enhance observation, attention, and memory.

Lesson task: To construct a regular polyhedron from cardboard using a dodecahedron net. This promotes the development of fine motor skills of the fingers, spatial imagination, geometric thinking, cognition, and creativity.

Practical work is conducted in groups. Visually impaired students are assisted by teachers or sighted children, using a magnifying glass to improve visibility. The workspace should be well-lit. Materials needed include cardboard, scissors, glue, and a dodecahedron net should be available on each student’s desk.

Before starting the practical work, there is an oral discussion about regular polyhedra.

Question: How many types of regular polyhedra exist?

Answer: There are five regular polyhedra.

Question: What are the regular polyhedra?

Answer: Tetrahedron, hexahedron, octahedron, icosahedron, and dodecahedron.

Question: Why do the names of regular polyhedra end with “hedron”?

Answer: The word “hedron” comes from the ancient Greek “hedra” meaning face.

Question: What equilateral shapes make up a dodecahedron?

Answer: Pentagons.

Question: How many faces does a dodecahedron have?

Answer: 12 faces.

Question: How many vertices does a dodecahedron have?

Answer: 20 vertices.

Question: How many edges does a dodecahedron have?

Answer: 30 edges.

On the board, there is an illustration of a dodecahedron.

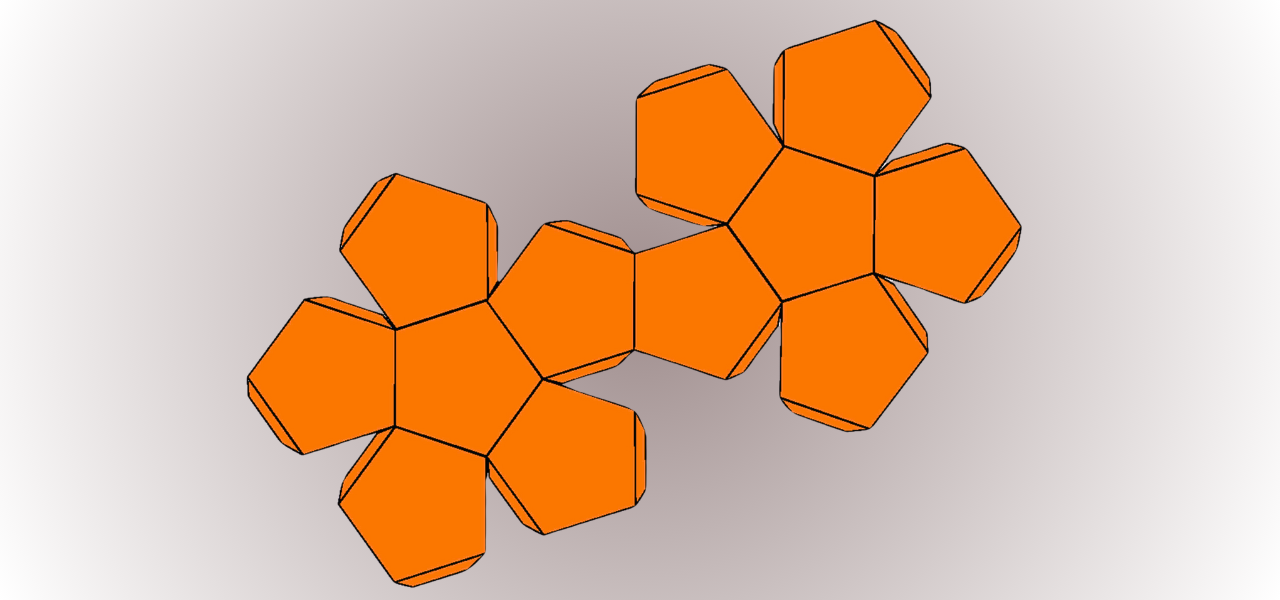
As a result of the work of several groups, models of dodecahedrons were created, which will serve as visual aids in geometry lessons. Examining these models and searching for objects that correspond to mathematical concepts stimulates the students’ cognitive activities and fosters the development of collective collaboration.

image: модель многогранника

Most often, when creating models of polyhedra from flat nets, these nets are designed so that the faces adjoin each other at the edges, and the model is formed by folding along these edges.

“The hand is the tool of tools,” Aristotle said, and this statement remains relevant today.