Developing Fine Motor Skills. Mathematics on the Fingers of the Hands
This material is recommended for high school students.
These methods may be useful for educators who teach or plan to teach mathematics to visually impaired students.
The first counting tool for ancient people was their own fingers. Counting on fingers was widely used in the ancient world and the Middle Ages, gradually improving and becoming more complex. Human fingers were not only the first counting device but also the first computing machine. Nature itself provided humans with this universal counting tool. For many peoples, fingers (or their joints) played the role of the first counting device in any trade operations.
In the lesson, we will try to understand how to use such a wonderful cheat sheet as our hands in our time.
One of the most difficult sections of mathematics in high school is “Trigonometry.” Students have difficulty navigating the intricacies of trigonometric functions. It is necessary to remember not only the formulas but also the values of the main angles, which seems difficult to memorize. To facilitate the memorization of the values of the angles of the first quadrant, let’s consider the open palm of the left hand.
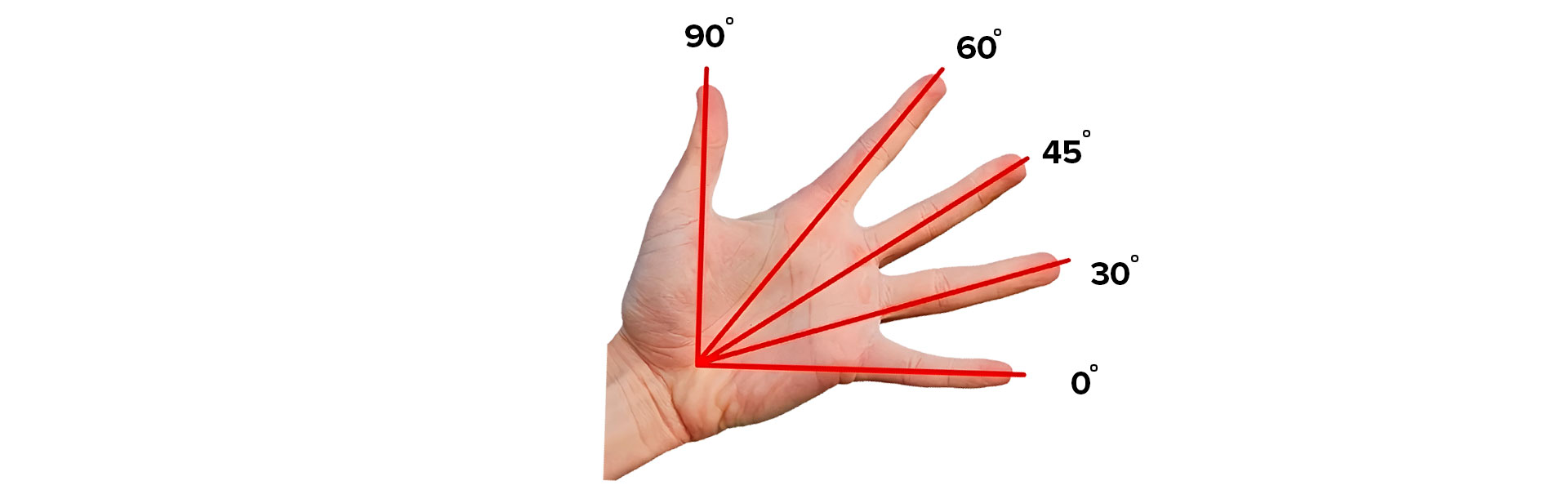
It turns out that the values of the sines and cosines of the angles are “located” in the palm.
Imagine lines drawn through the pinky and thumb. These lines intersect at a point called the “palm mound.” An angle of 90 degrees is formed. The pinky line forms a 0-degree angle. By drawing rays from the mound through the ring, middle, and index fingers, we obtain angles of 30, 45, and 60 degrees.
- Pinky (0 degrees),
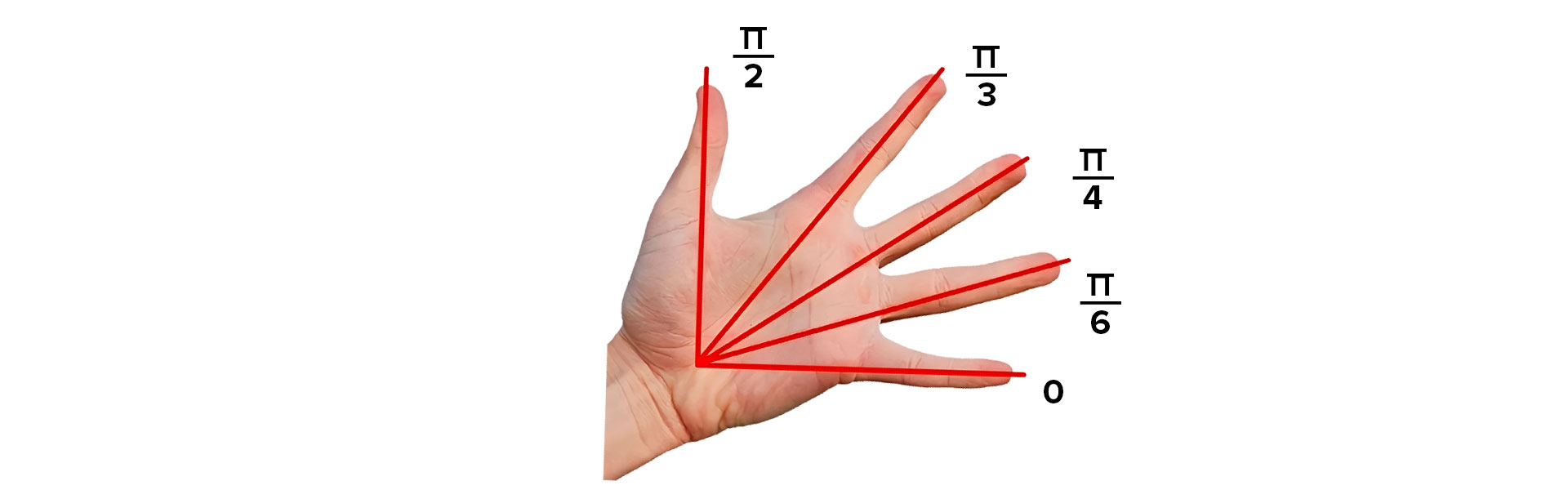
- Ring finger (30 degrees) or π/6,
- Middle finger (45 degrees) or π/4,
- Index finger (60 degrees) or π/3,
- Thumb (90 degrees) or π/2.
Using the palm, we remember the angles of the first quadrant. Then, remember the values of the angles of the first quadrant. It is necessary to know by heart the values of the trigonometric functions of the main angles. Along the x-axis are the values of the cosine, and along the y-axis are the values of the sine.
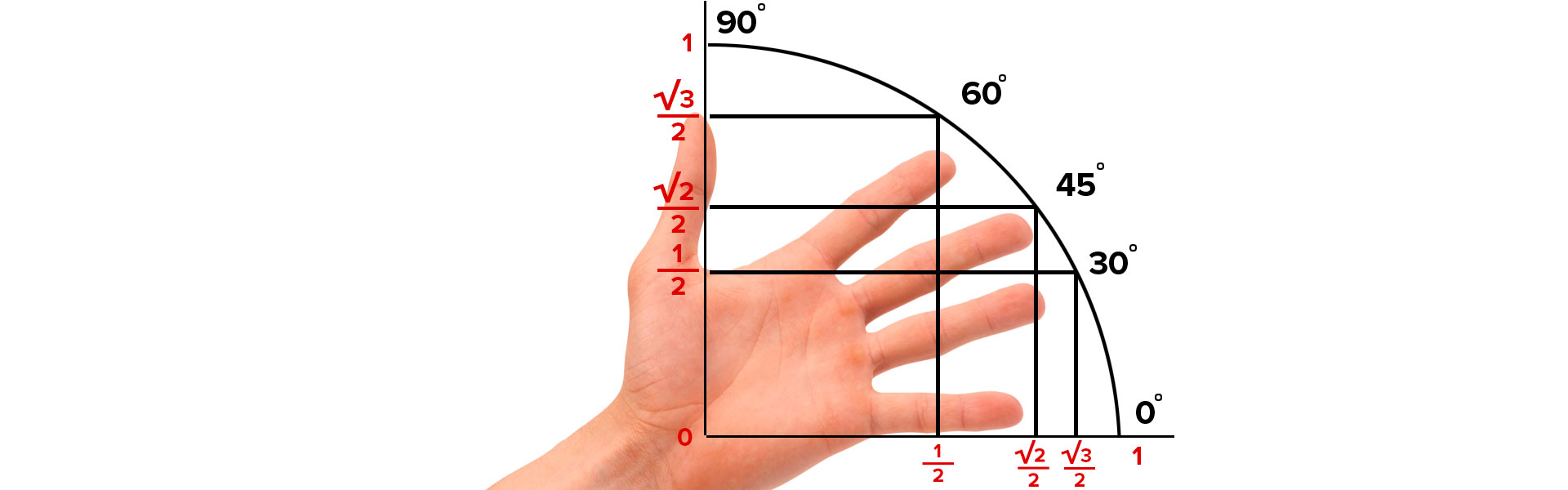
To memorize, you need to take the square root of the finger number and divide it by half to get the sine of the angle corresponding to that finger.
For example, if you need to find sin60°, it corresponds to the index finger, which is number 3.
To find the cosine, you should number the fingers in reverse order.
The use of mnemonic techniques in studying trigonometry is justified and necessary.
When teaching in a classroom with visually impaired students, it is important to learn to use various techniques that facilitate memorization and understanding of the material.